Rule 10: Reducing Fractions
Rule 10: Reducing Fractions
To reduce a simple fraction, follow the following three steps:
For example, reduce 
.
First: Rewrite the fraction with the numerator and the denominator factored.
Note all factors in the numerator and denominator are separated by multiplication signs.
Second: Find the fraction that equals 1. 
can be written 
which in turn can be written 
which in turn can be written 
.
Third: We have just illustrated that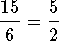 
. Although the left side of the equal
sign does not look identical to the right side of the equal sign, both
fractions are equivalent because they have the same value. Check it
with your calculator. 
and 
. This
proves that the fraction can be reduced
to the equivalent fraction .
Example 1: Reduce the fraction 
.
Answer. Factor the numerator and factor the denominator and look for the fractions in the mix that have a value of 1.
and
The fraction has been reduced into the equivalent
fraction 
.
Now prove to yourself with your calculator that both
fractions are equivalent. When you divide 120 by 180, you will get
the same answer as when you divide 2 by 3.
If you would like to see another example, click on the word
Example.
Work the following problems and click on Answer
to check your results.
Problem 1: Reduce the fraction 
.
Problem 2: Reduce the fraction 
.
Problem 3: Reduce the fraction 
.
Problem 4: Reduce the fraction 
.
Problem 5: Reduce the fraction 
.
S.O.S MATHematics home page Do you need more help? Please post your question on our S.O.S. Mathematics CyberBoard.
Author: Nancy Marcus