 INTEGRALS CONTAINING Coth(ax)
INTEGRALS CONTAINING Coth(ax) INTEGRALS CONTAINING Coth(ax)
INTEGRALS CONTAINING Coth(ax)

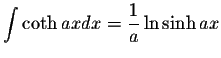
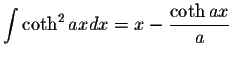
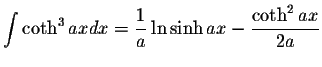
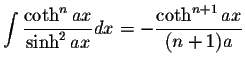
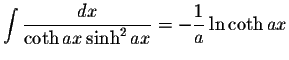
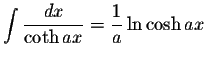
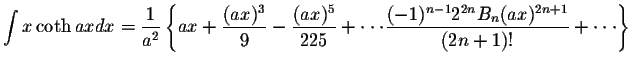
where the constants Bn are the Bernoulli's numbers.
where the constants
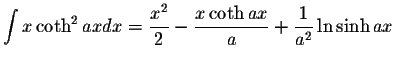
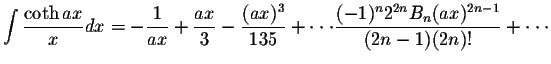
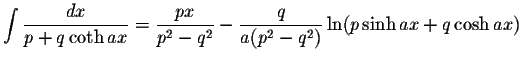
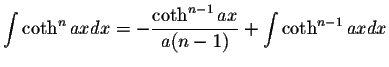

 S.O.S MATHematics home page
S.O.S MATHematics home page Do you need more help? Please post your question on our S.O.S. Mathematics CyberBoard.
