The Particular Case of Positive Series

Consider the series and its associated sequence of partial sums . Here we will assume that the numbers we are about to add are positive, that is, for any . It is clear that the process of generating the partial sums will lead to an increasing sequence, that is,

,

for any . Our previous knowledge about increasing sequences implies the following fundamental result:

The positive series is convergent, if and only if, the sequence of partial sums is bounded; that is, there exists a number M > 0 such that

,

for any .

This result has many implications. For example, we have the following result (called The Basic Comparison Test):

Consider the positive series .

1.

Assume there exists a convergent series such that

,

then the series is convergent.

2.

Assume there exists a divergent series such that

,

then the series is divergent.

Recall that in previous pages, we showed the following

,

which means that the series is divergent.

Example: Show that the series

is divergent.

Answer: We have , for any ; hence

.

Since is divergent, we deduce from the Basic Comparison Test, that 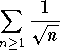is divergent.

Example: Show that the series

is convergent.

Answer: Since

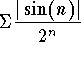and the geometric series is convergent, then the series is convergent (using the Basic Comparison Test).

The next result (known as The p-Test) is as fundamental as the previous ones. Usually we combine it with the previous ones or new ones to get the desired conclusion.

Consider the positive series (called the p-series) . Since the limit of the numbers must add to 0, in order to expect convergence, we assume that p > 0. The next result deals with convergence or divergence of the series

when p >0.

The p-series converges, if and only if, .

The proof of the above result is very instructive by itself. So let us discuss how this works:

Consider the function defined by

.

It is easy to check that f(x) is decreasing on . Hence, for any , we have for any ,

which implies

,

that is,

.

Using this inequality, we get

and

.

If , then we have

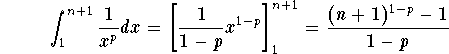
and if p=1, then we have

.

We have three cases:

Case 1: p < 1, then we have

.

Since 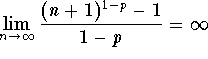, then the series is not bounded,and therefore it is divergent.

Case 2: p > 1, then we have

but, since

,

we get

,

which means that the sequence of partial sums associated to the series is bounded. Therefore, the series is convergent.

Case 3: p = 1, we have already shown that the series is divergent. Though one may want to easily check that we have

,

which shows that the sequence of partial sums is not bounded.

Example: Discuss the convergence or divergence of

.

Answer: It is not hard to show that for any , we have . Then, we have

.

Since, by the p-Test, the series is convergent, the Basic comparison Test implies that 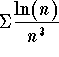is convergent.

The last result on positive series may be the most useful of all. Indeed, the Limit Test should be always in mind when it comes to cleaning up some undesirable terms.
Before we state this test, we need a new notation. Indeed, we will say that the two sequences and are equivalent, or , if and only if,

.

Let and be two positive series such that

.

Then converges, if and only if, converges.

Example: Determine whether the series

is convergent or not.

Answer: Note that when n is large we have and . Then it is easy to check that

.

Using the p-test we get that the series is convergent. Hence, by the Limit-test, we deduce the convergence of the series .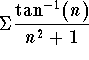

Example: Determine whether the series

is convergent or not.

Answer: We know that

.

This limit means that when x is very small , then (a very useful conclusion in physics, for example, when dealing the motion of the pendulum). So when n is large, 1/n will be small and therefore 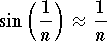. This clearly implies that

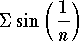Since the series is divergent, the limit-test implies that the series is divergent.

Remark: It should be appreciated that, without the Limit-test, it would be very hard to check the convergence.

[Trigonometry] [Calculus] [Geometry] [Algebra] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra]

S.O.S MATHematics home page

Do you need more help? Please post your question on our S.O.S. Mathematics CyberBoard.

Copyright � 1999-2024 MathMedics, LLC. All rights reserved.
Contact us
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA
users online during the last hour